Data
- Title: Asymptotics in finite monoidal categories
- Authors: Abel Lacabanne, Daniel Tubbenhauer and Pedro Vaz
- Status: Proc. Amer. Math. Soc. Ser. B 10 (2023), 398-412. Last update: Thu, 9 Nov 2023 08:10:44 UTC
- ArXiv link: https://arxiv.org/abs/2307.03044
- ArXiv version = 0.99 published version
- LaTex Beamer presentation: Slides
Abstract
We give explicit formulas for the asymptotic growth rate of the number of summands in tensor powers in certain monoidal categories with finitely many indecomposable objects, and related structures.
A few extra words
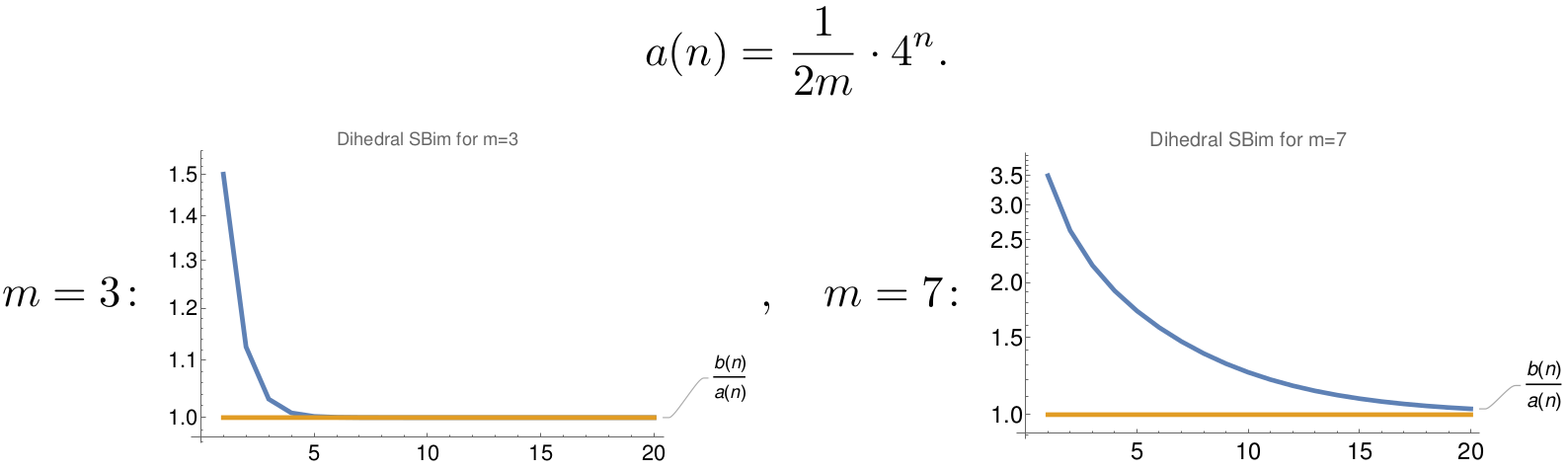
The main theorems of the note give an explicit formula \(a(n)\) to compute the asymptotic growth of summands
in tensor products \(b(n)\) for a large class of categories.
For example, for dihedral Soergel bimodules one gets: